Area of Parallelograms
Concept
A parallelogram is a special kind of quadrilateral that is formed by parallel lines. The term ‘parallelogram’ was derived from the Greek word ‘parallelogram on’ which stands for “bounded by parallel lines”. Hence, a parallelogram is a quadrilateral that is bounded by parallel lines. It is a shape in which the opposite sides are parallel and equal. Parallelograms are classified into three main types: square, rectangle, and rhombus.
The area of a parallelogram is defined as the region or space covered by a parallelogram in a two-dimensional plane. The area of a parallelogram refers to the total number of unit squares that can fit into it and it is measured in square units (like cm2, m2, in2, etc.).
Rules
To find the area of a parallelogram, multiply the base by the height.
Area = b × h (h is at right angles to b)
Example
Calculate the area of the parallelogram to the nearest tenth of a unit.
Solution
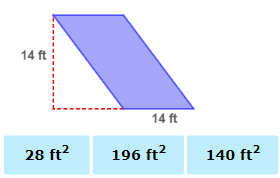
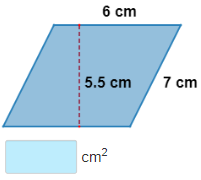
To find the area of a parallelogram, multiply the base by the height.
A = b × h (h is at right angles to b)
A = 2.5 m × 1.8 m
A = 4.5 m2
Practice Area of Parallelograms
Practice Problem 1
Calculate the area of the given parallelogram.
A = b × h
Practice Problem 2
The parallelogram below is on a centimeter grid. What is its area?
A = b × h
Practice Problem 3
Calculate the area of the parallelogram to the nearest tenth of a unit.
Practice Problem 4
Given the area, calculate the missing height or length of the parallelogram.
A parallelogram is a quadrilateral with opposite sides parallel and equal in length. Every parallelogram can be made into a rectangle, which is why we use the same formula to find the area of a parallelogram and a rectangle.
Pre-requisite Skills
Measurement – Perimeter
Measurement – Area
Classify Quadrilaterals
Quadrilateral Facts